Neutrinos are Undulations in the Charge Field
also the Solar Neutrino Problem
© Miles Mathis
There was a great fuss about experiments proving that neutrinos can travel faster than c but some prominent physicists are saying no. The arguments will not be discussed here since no one knows what they are talking about anyway. The flaw in this experiment is that it centers on the expectation that the
neutrinos should lose energy as they travel the path. This just proves that mainstream physicists do not
know what neutrinos are. All along, particle physicists have assumed hat neutrinos are particles
somewhat like photons, with zero rest mass but a tiny energy. The neutrino came from beta decay
experiments, and it was proposed by Wolfgang Pauli in 1930 to fill energy gaps there. Very little has
changed since then. Unfortunately, the gap is not properly explained by a new particle, as MM has shown; it is caused by magnetic or spin change to the surrounding charge field, which causes a small energy
change. This change is localized, and therefore it acts somewhat like a particle, but it is a field wave,
like sound. Again, a field wave, like sound; not the spin of a particle, like light. This localized field
change can then travel through the charge field at the speed of light, still acting somewhat like a
photon, but it neither a photon nor a neutrino. It is not a particle at all. It is a wave like sound, which
exists only as a pattern on a background. This is precisely why their neutrino in this new experiment
is not acting like a particle, losing energy as it moves through a field. This charge field wave wouldn't be
expected to lose energy like a particle, it would be expected to lose energy like a wave. But since they
are applying particle equations to it instead of wave equations, they get the wrong answer.
One might sat that they are applying quantum equations, which are wave equations, but this is not the case. They are applying quantum equations, which are titled wave equations, but which are not really field
wave equations. Quantum equations were invented to apply to quanta like photons and electrons,
which are particles. The equations have been tweaked over the years to work on those particles, so it
does not matter what we call them, they are still particle equations.”
The mainstream believes that quanta have both wave and particle qualities and the quantum equations also have particle and wave qualities, but quanta are just real particles. These wave characteristics
are not equivalent to field wave characteristics. You see, the mainstream has not yet discovered that we
have two sorts of wave equations: field wave equations and spin equations. The spin equations mirror
wave equations in many ways, since the spins create waves, but fundamentally they are not the same.
A wave created by spin will not travel exactly like a wave created by a field disturbance. This is because
a spin is not just a field disturbance. Spin is the motion of a real particle, and so it travels with the
particle. A field wave does not travel with the particle, so the paths of the two waves will not be the
same. In short, the spin wave will diminish slightly faster than the field wave, and this is due to the
nature of collisions in the field. Both waves propagate via collisions in the field, but they propagate in
fundamentally different ways. Individual photons will lose more energy as they move through a field
than will a field wave, and this is because photons lose energy in collision, while a field wave transmits
energy in collision. A field wave will lose some energy, but it loses less than a spin wave.
To see in more detail why this is, we have to study the collisions in the field carefully. If you have a
photon moving through a charge field, you have photons colliding with other photons. The charge field
is photons. But since all photons are spinning, collisions affect only the outer spins. Photons are
interpenetrable to a photon field to a pretty large degree, but when we do have a collision, we find only
a spin damping. We get edge to edge hits, and the spins are affected mechanically. The spins offset,
like little gears, and the free photon loses a little energy and the charge photon gain a bit. That is how a
spin wave is damped.
However, if we have a field wave moving through the charge field, we have a slightly different mechanics.
To see this, we have to return to the mechanics of beta decay. A positron impacts a neutron, reversing
the outer spins of both. By reversing the outer spin, the positron becomes an electron. The positron
was emitting an anti-photonic charge field, but the new electron is emitting a photonic charge field.
Due only to spin, the charge field of anti-matter is upside-down compared to matter.
Since the
ambient charge field is photonic, the ambient field gets a boost. However, this field boost, though localized,
is not completely localized. Meaning, it is not localized to one particle. The new electron is emitting a lot
of charge photons, and all these photons interact with the ambient field. All of these charge photons
bump other charge photons, transferring energy via spins, just like the free photon did. But since we
are tracking the field now, instead of the individual photon, we do not see the same sort of damping.
If we track the spin on one photon, then that spin will diminish as we travel through the field. At the
end, we sum all those free photons, to find the total diminishment. But if we track the field wave, we
are still tracking individual collisions, but we are switching particles after each collision. In other
words, we have a collision, and the first photon transfers energy to the second. But if we are tracking
the field wave, we dump the first photon and now follow the second. The second has increased its
energy, not decreased it. So if we track the field energy, we see it moving across the field with very
little diminishment. From a certain point of view, it will look like a particle moving through the field.
That is what a neutrino is.
The photon is a particle with spin, and the spin causes the appearance of a wave. The neutrino is not a
particle. The neutrino is a definite energy change moving across or through a charge field. The
neutrino may look like a particle, but it is not. And its energy diminishes much less than the photon's
energy diminishes, as they both travel through a field. In fact, this is the best way to figure out if you
are dealing with photons or neutrinos. Neutrinos will travel with little or no diminishment through
charge fields, and even through matter. This is known, but here is the explanation using simple mechanics./p>
First published March 28, 2013
After being a mystery for decades, this problem was said to have been solved a few years ago. In this
way, it is somewhat like the Moon's ionosphere problem on MM's site , now claimed to be solved by moon dust. MM proved this to be wrong as he will with this problem.
It has been known since the late 1960's that the number of neutrinos produced by the Sun is 66% too
low. The Sun is producing only 1/3rd the number expected, according to mainstream measurements.
To explain this big miss in prediction, particle physicists decided to give the previously massless
neutrino a small mass. They also claimed that the neutrino could change forms, from an electron
neutrino to a muon neutrino or tau neutrino. If 2/3rds of electron neutrinos were turned into other types
between here and the Sun, the problem would be solved.
Before we get to the specifics of this terrible theory, just notice how illogical it is from the beginning.
We are told that most neutrinos pass through the Earth with no effect. Less than 1% suffer life-
changing collisions when passing through thousands of miles of dense material. But we are supposed
to believe that 66% of neutrinos suffer life-changing collisions or interactions when passing through the
nearly “empty” space between here and the Sun? Talk about a theory with no legs, that refutes itself
from the first word.
Since MM has shown that precisely none of these particles actually exist, he knows this solution is another
terrible fudge. First, MM showed in A Reworking of Quantum Chromodynamics and dismissal of the Quark that the so-called electron neutrino was proposed to fill a hole in beta
decay, but since this hole is just a charge-field lack of symmetry, it shouldn't be filled with a particle.
It is simply the difference between the ambient charge field before and after the decay, and it is only a
spin difference, not a particle difference.
In other words, in beta decay, the neutron does not decay at all.
It gets hit by a positron. The outer spins of both particles reverse, turning the neutron into a proton and
the positron into an electron. Since all this is happening in an ambient charge field, and since this
ambient field has a summed spin, the summed spin of the ambient field will change very slightly
locally due to the hit.
The incoming positron was negative to the field and subtracted a small amount
of spin from it, but the electron does the opposite. Instead, a small amount of spin is added to the
ambient field total. Or, the positron was pouring antiphotons into the field, but the electron is pouring
photons into the field. Locally, the summed spin of the ambient field has changed. This spin change is
what we have called the neutrino. Since the spin change is localized—it is localized to the spot where
the hit took place—it will appear to be a particle. It will also travel. It moves away from the spot of
the hit, with a definite direction determined by the hit. So again it may appear to move like a particle.
But it is not a particle, it is a field wave. In this way, it is analogous to a water wave, which is also a
field wave. The wave in water may act like a particle in some ways, but it is not. Just so, the electron
neutrino.
MM has explained the older experiments mechanically, but what of the newer experiments that
claim to discover the proof of neutrino oscillation? Here they have find all three neutrinos, believing that the
numbers from the Sun have been verified? However, MM will show that the data is easier to read as proof of his charge field.
To start with, they tell you that 2/3rds of the total electron neutrino emission turns to muon neutrinos or
tau neutrinos between here and the Sun, but they have no evidence of that. Meaning, they do not have
any proof or indication that those “oscillations” are actually happening. We do not have any counters
parked in space around Venus, for instance, monitoring electron neutrinos going in and muon or tau
neutrinos going out. The only evidence we have is from SNOLAB and a couple of other places, which
claim to find the various neutrinos in the right amounts here on Earth. But how would we know that
those muon neutrinos and tau neutrinos at SNOLAB are the ones that oscillated on the way here from
the Sun? The Sun is not the only producer of either. The page at Wikipedia admits muon neutrinos are
produced in the upper atmosphere by cosmic rays.
The physicists at SNOLAB are extrapolating from very little data. They get very few hits, which was
the whole point of going 2km down into the Earth. Do they know where their particles are coming
from? No. They have no way of knowing if the muon neutrinos they claim to detect are coming from
the Sun, via oscillation, or from the upper atmosphere, via cosmic rays.
To see the state of the art, let us look at a quote: The first strong evidence for neutrino oscillation came in 1998 from the Super-Kamiokande collaboration in Japan.
It produced observations consistent with muon-neutrinos (produced in the upper atmosphere by cosmic rays)
changing into tau-neutrinos. What was proved was that fewer neutrinos were detected coming through the Earth
than could be detected coming directly above the detector. Not only that, their observations only concerned muon
neutrinos coming from the interaction of cosmic rays with the Earth's atmosphere. No tau neutrinos were observed
at Super-Kamiokande.
Please note that this paper is a simplification by me of a paper or papers written and copyrighted by Miles Mathis on his site. I have replaced "I" and "my" with "MM" to show that he is talking. All links within the papers, not yet simplified, are linked directly to the Miles Mathis site and will appear in another tab. (It will be clear which of these are Miles Mathis originals because they will be still contain "I" and "my".) The original papers on his site are the ultimate and correct source. All contributions to his papers and ordering of his books should be made on his site.
(This paper incorporates Miles Mathis' neut2 paper,
snp paper, and
spiral paper.)

Pauli
The Solar Neutrino Problem
In the above, notice that sentence two tells you this experiment produced observations consistent with muon neutrinos turning into tau neutrinos. Then notice the last sentence: “No tau neutrinos were observed.” If no tau neutrinos were observed, then what “observations” indicated they were produced? They think the muon neutrinos were turning into them with any basis yet they think that has solved this problem! with this “solved problem.”
The convincing evidence for solar neutrino oscillation came in 2001 from the Sudbury Neutrino Observatory (SNO) in Canada. It detected all types of neutrinos coming from the Sun, and was able to distinguish between electron- neutrinos and the other two flavors (but could not distinguish the muon and tau flavors), by uniquely using heavy water as the detection medium. After extensive statistical analysis, it was found that about 35% of the arriving solar neutrinos are electron-neutrinos, with the others being muon- or tau-neutrinos.
Does that sound convincing to you? We have an experiment that admits it cannot even distinguish between two of the three particles being studied, and yet we are supposed to have proof of a solution? Apparently all this experiment at SNOLAB found was about 1/3rd electron neutrinos. But we have known that since 1968. The 2002 Nobel Prize went to the guys that found that deficit in 1968. So I think we need more than that.
If we dig a bit deeper, it starts to get really ugly:
As a neutrino propagates through space, the quantum mechanical phases of the three mass states advance at
slightly different rates due to the slight differences in the neutrino masses. This results in a changing mixture of
mass states as the neutrino travels, but a different mixture of mass states corresponds to a different mixture of
flavor states. So a neutrino born as, say, an electron neutrino will be some mixture of electron, mu, and tau
neutrino after traveling some distance.
Clearly, this does not sound like physics to me. It seems like a big fudge. When they start saying stuff like that, you can be sure they do not know what they are talking about. They are just making it up as they go. Ask them if they have any evidence or data for that statement. No. How could they? What possible data could there be? Have they ever found a mixed state particle? What would a mixed state particle look like, to a detector? Would it have three different masses or energies? Sounds like it would just be a blob that they couldn't distinguish, as above with their data at SNOLAB. They must be selling their inability to distinguish the tau neutrino from the muon neutrino as proof that they caught the particle in mid-oscillation. “It was in the process of oscillating, so we couldn't get a get grip on it.” In this way, any blobby indistinct data can be used as proof of a theory. The worse your data set is, the better you will like it. “Our data set has no resolution, no sigma, and is indistinguishable from background, so yippy, let's celebrate. It must be proof of a mixed eigenstate!”
But let's continue to pick apart their text. They make it so easy:
The idea of neutrino oscillation was first put forward in 1957 by Bruno Pontecorvo, who proposed that neutrino-
antineutrino transitions may occur in analogy with neutral kaon mixing. Although such matter-antimatter oscillation
has not been observed, this idea formed the conceptual foundation for the quantitative theory of neutrino flavor
oscillation, which was first developed by Maki, Nakagawa, and Sakata in 1962 and further elaborated by
Pontecorvo in 1967. One year later the solar neutrino deficit was first observed and that was followed by the
famous paper of Gribov and Pontecorvo published in 1969 titled "Neutrino astronomy and lepton charge".
They really let it all hang out, do not they? Although the proposed transitions have not been observed to this day, they decided to base a whole theory on it. Strange that they admit the matter-antimatter oscillations have not been observed, but do not admit it about the neutrino oscillations. Notice they had the theory before they had the problem. This oscillation theory had been sitting around since 1957. By 1967, it had morphed into neutrino oscillation, and the very next year they found a problem they could solve with it. It was a prize just waiting to happen.
This is how new physics works. They do not have a problem first, and then a solution. No, they have solutions just waiting around. The mathematicians have fudged equations into the next century, and the physicists are just sniffing around for problems to apply them to. And they admit this, in public, on the 6th largest website in the world. And why 2/3rds? Why not half? Why not .61? They do not have a simple, logical explanation for the split. MM's simple logical explanation for that number, is shown below.
That 1/3td, 2/3td split is our first clue. The second clue is in the fact that the standard model thinks all neutrinos are left-handed. Although it has both neutrinos and anti-neutrinos, it does not give that split to chirality or spin. Actually, there is some confusion on this point, since the neutrino page at Wikipedia tells us the split is due to chirality, but the neutrino oscillation page tells us its is not. On Raymond Davis' page, it tells us that “detecting neutrinos proved considerably more difficult than not detecting antineutrinos.” So we have obviously detected a lot of anti-neutrinos. If they are telling us that right-handed neutrinos have not been found, they must be telling us that anti-neutrinos are not right-handed. Which means that the “anti” tag is not telling us chirality.
The reason neutrino theory is such a godawful mess—despite all the work done on it—is that the standard model has refused to this day to give the photon real mass, real radius, and real spin. They do not have photons and antiphotons to work with, and this has doomed all neutrino theory from the start. There are some limited theories use antiphotons, but even then they do not give the particles real spin. They just give them more unassigned quantum numbers, which only adds to the mess.
The next section on pair production will help to explain where the 1/3rd, 2/3rd split comes from. This paper explains why the charge field on the Earth is split 1/3rd, 2/3rd.
A Better Theory of Pair Production
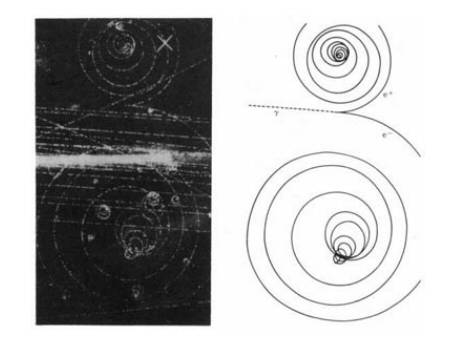
The image above is a famous result from a particle accelerator [Blackett, 1933], supposedly showing the creation of an electron-positron pair. As you see, the positron has a smaller and tighter spiral than the electron. If the particles are equal in mass and opposite in charge, why aren't the spirals the same? The standard model has no way to explain it, so they ignore it. It is doubtful you have ever seen the question addressed, and it is doubtful you have ever asked the question yourself. Yet, this is actually a confirmation of MM's charge field theory.
In explaining beta decay in A Reworking of Quantum Chromodynamics and dismissal of the Quark, MM proposed that the current theory is wrong. It is known that beta decay is not symmetrical, and this is currently explained with weak theory, symmetry breaking, borrowing from the vacuum, pushing quark flavor and color, and all sorts of other wickedness. But I showed that it was much simpler than that. The expectation that the field must be symmetrical is simply a false expectation. The charge field here on the Earth is not symmetrical, because it has more photons than anti-photons. That is why the Earth is very magnetic, while Mars and Venus and the Moon are not. Any magnetic field will be non-symmetrical regarding photon spins, since the excess spins are what underlies magnetism. Only a field like that of Venus is nearly symmetrical. So there is no rule requiring local spin symmetry on the charge field. The rule of symmetry is only global (universal).
We have another problem with the image above, and that concerns the quick loss of energy of both quickly. We might understand why the positron is losing energy: if it is being emitted into a field of photons spinning opposite to it, it would logically lose spin energy and continue to decay. But that doesn't explain the electron doing the same thing, at a slightly slower rate. If the ambient charge field doesn't support the positron, it should at least support the electron. We should see the electron move off in a straight line, and even gain energy. Why don't we?
It is because nothing we have been told is true. It isn't a photon that is decaying into an electron and positron. It is a tau neutrino decaying into two electrons and two positrons. We don't see the other two particles only because they are moving directly toward or away from us. The image isn't set up to track particles in four directions or 360 degrees. Since we are viewing these two spirals straight to the side, the other particles won't leave visible tracks in the image.
In Explaining Mesons without Quarks, MM showed that the tau neutrino is not really a neutrino. We have called a lot of different things neutrinos, and we now need to separate them and distinguish between them. What we normally call a neutrino is not a particle at all. It is just a field wave in the charge field. Then we have the muon neutrino, which MM has shown is just three non-spinning electrons huddling for protection from the charge wind.
The tau neutrino is four x-spinning electrons, brought together by a very specific reaction or interaction. Once that interaction is finished, the electrons will split again, and that is what we are seeing here.
MM can even show you how the tau neutrino is created. The most recent example was created in 2008 by
the Titan laser being shot at a gold target. We are told that many electron-positron pairs were produced,
and that is true. But the pairs were produced in pairs, like this:
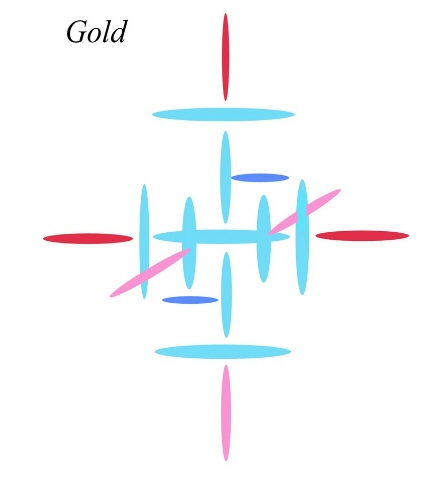
That is the gold nucleus.
(Other elements are shown in How Elements are Built - A Mechanical Explanation of the Periodic Table)
MM has shown that electrons don't orbit the nucleus, they orbit a charge minimum at the pole of the proton. Each of the colored disks represents some number of alphas, with two protons to each alpha. So the electrons are in the disks. When we fire a laser at an atom, it works preferentially on the outermost level of the nucleus, which in my diagram would be the red and purple disks. This is the fourth level, which in some ways corresponds to the fourth electron level in current theory. In this case, we will look at the carousel level of the gold nucleus, which is the four red and purple equatorial disks here. If we let this nucleus spin like the Earth, about a north-south axis, these four red and purple disks will be spinning like a carousel, you see. To watch this motion, you may look at Steven Smith's animation of gold . He also diagrams the electrons, so this may help you.
At any rate, when the laser beam falls on this gold nucleus, it does so in a line. The line of the beam intersects the plane of the carousel spin, and if the beam is aimed right, it begins knocking the electrons out of the disks. Because we have a line meeting a plane, all the electrons are knocked out in the same plane. Keep that in mind as we pull in another new piece of information.
As these electrons are knocked out of the nucleus, they are randomized according to spin. In other words, the laser may hit some electrons above center and some below. Therefore, some free electrons will be bumped out rightside up, and some will be bumped out upside down. Since, in most cases, an upside down electron is equivalent to a positron, the laser will be turning half our electrons into positrons, simply by flipping them over. This is the basic mechanism of pair production.
But then why would the electrons come together even for a short time in pairs or fours? The mainstream can't explain that (they haven't really tried), so they are forced to assume that photons decay into the pairs. Well, we have already brought the electrons into the same plane, so we are halfway there. A beam in a line ejecting electrons from the same plane will eject them into the same plane. Then we look at the ambient charge field. The experiment will be done in some ambient charge field. Unless the charge is moving in the same direction as the laser beam (which is very unlikely, as a matter of chance), this charge field will act as a second focus on the ejected electrons. The laser will be pushing them in one line and the charge field will be pushing them in a second, different line. This creates a focus. The electrons and positrons are brought together.
Still doesn't explain the four, you will say. It does, because the carousel level of gold has four disks, as you see. The carousel level is four disks in a plane, with all disks orthogonal to the others. That is precisely what we find in the tau neutrino and the bubble chamber spirals: four particles orthogonal in the same plane. As the laser interacts with the electrons in gold, it bumps four electrons out simultaneously, and the electrons are already orthogonal and in the same plane. Problem solved.
Now let us look at the electrons themselves. MM has shown that they are x-spinning electrons. What does that mean? It means that the laser has transferred energy to them in a quantized way, so that they are no longer normal electrons. They actually have 7.2 times the spin energy of a normal electron. In bumping the electron out of the nucleus, the laser photon has stacked an extra spin on top of the electron. The laser photon loses the spin energy and the electron gains it. This also gives the electron a greater radius, since we measure the new spin radius. According to my quantum spin equations, the electron is really a first level meson. It is a spin level above the electron. If we stacked another spin on it, it would be a muon, and if we stacked another on top of that, it would be a baryon. In other words, there are two primary levels of meson, and our x-spinning electron is now temporarily inhabiting the first level.
And this is why it must spiral down. What we are seeing in the image under title is the x-spinning electron spiraling down to the normal electron. The normal electron has only an axial spin, but no x- spin. We are watching the electron lose about 86% of its energy, going from 7.2 to 1.
You will ask why it can't keep the energy it got from the laser. Why isn't the x-spinning electron stable? It isn't stable because the electron gets all its energy from the field. All quanta are continuously energized by charge. After it is ejected, the electron is no longer existing in the laser beam. It is once again existing in the ambient field. The ambient field can't keep the electron energized at its new 7.2 level. The electron exists by recycling the ambient charge field, and the ambient charge field isn't as energetic as the laser. Therefore the electron must fall back down to the level of the field.
But now we return to our first question: why is the positron spiral smaller than the electron spiral? It is the ambient charge field again, which MM has shown is not symmetrical here on Earth. If we ran this experiment on Venus, the spirals would be more equal. But here, where we have a strong magnetic field, the charge field is not balanced. It contains more photons than anti-photons, and this ambient spin supports the electron better than the positron. As soon as the positron hits the ambient field, it is squashed by the ambient charge field, because this field of photons is spinning opposite to the positron. We can even measure the ambient field by measuring the spirals. Notice that the electron spiral is exactly twice as big as the positron spiral. That means there are twice as many photons in the field as anti-photons. That is pretty extraordinary, as a matter of number, and we will look at that more closely in future papers.
In closing, there is one more extraordinary thing. We are told that everything is quantized at the quantum level, but we have clear evidence here that it isn't. Some things are quantized, some things aren't. To create this spiral, the electron must be losing energy in a continuous manner. A spiral is not quantized. It is a continuous curve. It is physically impossible to draw a spiral like this in a bubble chamber with a quantized curve. There is no such thing as a quantized curve. For the electron to lose energy in a quantized manner, it would have to emit a photon or something. But we don't see that here. The electron doesn't shed that extra spin level all at once, does it? It loses spin just like you would if someone stopped pushing your carousel. What this means is that the electron tends to gain energy in a quantized manner, because the photons spins are quantized. But it can lose energy in a continuous manner. The spin slows in a natural manner, and there is no need for the electron to emit anything to slow this spin. Yes, the beginning and end points of this spin loss are quantized, since the electron moves to definite levels which are supported by the charge field. But the electron does not have to jump instantaneously from one spin level to the other in going down, as we see clear evidence of here. These spirals are rather obvious data against Bohr's quantum leap.
Considering that these spirals are in a 2 to 1 ratio, which is the same as 2/3rd>, 1/3rd>. If we have twice as many photons as antiphotons, then in the total field we will have 2/3rd> photons. Simple math. Both the electron and positron are spiraling out in a field of charge, and if that charge field is split, then the electron will spiral larger than the positron. The positron is spiraling out in an opposing field of spins, while the electron is not. Real spins, real collisions.
These numbers relate well to the Solar Neutrino Problem also explain why the establishment thinks all neutrinos are left-handed. Since electron neutrinos are actually field waves, they have to travel as field waves. Which means they are just blips or humps in the ambient charge field. They are not real particles. Since the ambient charge field on the Earth always sums to photon, not antiphoton, the total field will always be measured as photon. Although 1/3rd> of the field. Since there will always be more photons in even the tiniest space, the field will always be measured to be photon, or left. This is why they think all neutrinos are left. Since the neutrino is a hump in the field, and the field always sums left on the Earth, any neutrino measured on the Earth will look left.
So why do we know about both neutrinos and anti-neutrinos? If the anti-neutrino is not right-handed, what is it? It is a spin deficit. In beta decay and anti-beta decay, we have different spin interactions. In the first, we have a positron flipping its outer spin to become an electron. As a positron, it was emitting anti-photons. As an electron, it is emitting photons. So at the point of the hit, we have small left-augmentation. We have a localized loss of antiphotons and a gain of photons. This may look like a particle to detectors, because it is so localized, but it is really something like a photon density increase in one little hump. In the second case, we have an electron flipping its outer spin to become a positron, with the opposite field outcome. Instead of a little hump of dense photons, we have a little hump of antiphotons, which can then travel out through the field. Problem is, our detectors cannot distinguish a photon field hump from an antiphoton field hump. Since both humps are travelling in a photon field, the detector will read both as left. The detector can't read the spin on the hump, it can only read the energy of the hump.
So the answer is that we know of anti-neutrinos both from the initial hit, which can be beta or anti-beta, and from subsequent reactions of the hump. We just can't directly measure the chirality.
Which brings us to the data from SNOLAB and other experiments. Since they are finding 1/3rd> of their total neutrinos to be electron neutrinos, we may infer that these are actually humps of anti-photons. Since they are traveling in a photon field, the detectors can only detect them as left, but they are not left locally. They are right. So why are they failing to detect the photon humps? Simply because the detector is using a magnetic detection. To make any kind of magnetic detection of the field, the detector has to be set to detect a certain configuration. In other words, the same detector can't detect both right and left at the same time. Why? Because the detector itself has to have one orientation or the other. The detector can't be both right magnetic and left magnetic at the same time. The detector is like a field itself, and we then measure the incoming particles relative to that set field. Well, the detecting field must either sum left or right. It can't sum both left and right at the same time.
This is what caused the initial problem back in 1968. Not realizing the nature of the ambient field, Ray Davis didn't realize neutrinos were traveling field humps in the photon field. He also didn't realize the ambient field of charge photons was already skewed 1/3rd> to 2/3rd> in the vicinity of the Earth. Since beta decay had already been misread when he came along, he just followed the conclusions of that theory, which were badly garbled. Everyone expected symmetry when there was no symmetry. Symmetry only applies globally, and the field of the Earth is a local field. There is no requirement of symmetry in a local field. Therefore, when he found he was detecting only 1/3rd> of the expected neutrinos, it looked like there was a big problem. But there never was a problem. We never needed oscillation. Even today, SNOLAB is not detecting oscillation, it may be detecting the elusive photon humps. We do not really know.
Remember, it is only finding two classes of particles, not three. It is finding electron neutrinos and muon/tau neutrinos, but it cannot discern between the last two. That is two classes, not three. Which implies it is probably detecting neutrinos and anti-neutrinos, not muon or tau neutrinos. If one of its two classes of detections is much larger than the other, it is probably because the machine does not have enough focus to detect the photon hump in the field of photons. It is therefore mistaking the left electron neutrino for the muon neutrino.
It is easier to detect an antiphoton hump in a field of photons, since it is easier to tell where the boundary is. The field gives you the boundary itself, and your detector does not have to create it. The “particle” has a more definite edge. But the hump of photons in a field of photons is like a glass of water thrown into the ocean. You can't tell where the hump ends and the field begins. If your detector is detecting a cross section that is slightly too large, you are going to be measuring the field beyond the hump, you see. Your particle is going to look bigger or more energetic than it actually is.
Conclusion
There was never any Solar neutrino deficit to begin with. The detectors were only detecting the right neutrinos, and since the right neutrinos are 1/3rd of the field, they were detecting just the right amount. Which means that neutrino oscillation is just one more embarrassing fudge that will soon have to be ejected from the textbooks and encyclopedias. The whole neutrino problem wasn't solved by oscillation, it has now been solved by MM's charge field.
The fact that neutrinos can travel through miles of dense material and photons cannot should be the clue that neutrinos are not particles. Neutrinos should be thought of as collections of particles or as shapes in a field of particles. With either definition, we would expect photons to interact differently in dense matter because that is what particles do. That is part of the definition of “particle.”
The only way neutrinos could have been particles and interact with matter as little as they do, is if neutrinos were billions of times smaller than photons. But they tell us the photon is already a point. How can you be billions of times smaller than a point? The photon has zero mass. How can a neutrino be billions of times smaller than a point with no mass? Even if we use MM's mass and radius for the photon, the neutrino would have to have a mass of something like 10-46 kg and a radius a billion billion times smaller than the proton.
We can think of the neutrino as either a shape or a group. If it is a shape, then any collision with matter will only knock out part of its shape. The rest of its shape can continue on. But even this is not a perfect visualization. Because the neutrino is a shape, not a particle, it won't collide with matter like a particle. A particle cannot be compressed, but a shape can. The shape has some field density and, as the neutrino shape travels through matter, the shape can compress or expand slightly. This allows it to dodge most collisions. The shape also does not have spin of its own. It may be a collection of internal spins, as we saw above, but it does not have a total edge spin, like any real particle would have. In other words, the neutrino is not a spinning sphere, with some sort of z-spin. Since almost all collisions of photons are edge hits, photons meet z-spin to z-spin. But neutrinos never collide in that way. They are not spheres and do not have z-spins. Only the outer part of the group or shape gets jostled, and in most cases we may assume this hit is not fatal to the whole shape or group.
For instance, say we let the neutrino be a dense collection of very small, very low energy photons. This collection of photons will not act like one larger photon. At the worst, an edge hit will strip off some of the photons on that side, but the rest will continue on. In that case, our neutrino may have lost a small part of its mass/energy, but it will continue on with no loss of velocity.
In sum, field shapes or waves are not as susceptible to total redirection as particles, and this is clear no matter how we think of them. It must be much harder to redirect a field hump than a particle, whether you think of the hump as a density, a shape, or a collection. In the end, this is because the particle acts like a structured solid, which cannot be easily split. Although these quantum particles are composed of stacked spins, and the spins can be stripped and augmented, the spins still have structure. Hitting a spin will effect the whole particle, simply because the whole particle is spinning . But a field hump acts more like a liquid. The constituent parts of the hump are more loosely bound. Edges of the hump can be compressed or stripped without effecting the whole hump. So while the photon moves through matter like a solid, the neutrino moves through matter more like a liquid or gas.
We can use water as an analogy here again. Since the neutrino is a field wave, we can think of the photon as like a water molecule, and the neutrino as like a water wave. If we put a rock in the stream, then send a water molecule right at the rock, the rock will hit it. We have a definite collision, and real stoppage or redirection. But if we do the same with the wave, the wave can go right around the rock, perhaps even rebuilding the lost segment on the other side. This is because the wave is only a shape. It is not a particle.